8.3 Payout Annuities
In the last section you learned about annuities. In an annuity, you start with nothing, put money into an account on a regular basis, and end up with money in your account.
In this section, we will learn about a variation called a Payout Annuity. With a payout annuity, you start with money in the account, and pull money out of the account on a regular basis. Any remaining money in the account earns interest. After a fixed amount of time, the account will end up empty.
Payout annuities are typically used after retirement. Perhaps you have saved $500,000 for retirement, and want to take money out of the account each month to live on. You want the money to last you 20 years. This is a payout annuity. The formula is derived in a similar way as we did for savings annuities. The details are omitted here.
Payout Annuity Formula
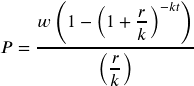
 is the balance in the account at the beginning (starting amount, present value or principal)
is the balance in the account at the beginning (starting amount, present value or principal)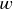 is the regular withdrawal (the amount you take out each year, each month, etc.)
is the regular withdrawal (the amount you take out each year, each month, etc.) is the annual interest rate (in decimal form for this formula)
is the annual interest rate (in decimal form for this formula)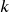 is the number of compounding periods in one year
is the number of compounding periods in one year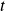 is the number of years we plan to take withdrawals
is the number of years we plan to take withdrawals
When to use this
Payout annuities assume that you take money from the account on a regular schedule (every month, year, quarter, etc.) and let the rest sit there earning interest.
Compound interest: One deposit
Annuity: Many deposits.
Payout Annuity: Many withdrawals
Example of a Withdrawal Annuity
After retiring, you want to be able to take $1000 every month for a total of 20 years from your retirement account. The account earns 6% interest. How much will you need in your account when you retire?
In this example,
![]() the monthly withdrawal
the monthly withdrawal
![]() 6% annual rate
6% annual rate
![]() since we’re doing monthly withdrawals, we’ll compound monthly
since we’re doing monthly withdrawals, we’ll compound monthly
![]() since we’re taking withdrawals for 20 years
since we’re taking withdrawals for 20 years
We’re looking for P; how much money needs to be in the account at the beginning.
Putting this into the equation:

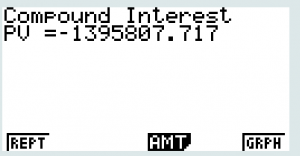
You will need to have $139,699 in your account when you retire.
Notice that you withdrew a total of $240,000 ($1000 a month for 240 months). The difference between what you pulled out and what you started with is the interest earned. In this case it is $240,000 – $139,600 = $100,400 in interest.
Using Technology
Similar to the annuities in the last section, we can use TVM Solver on a calculator or Excel to solve these problems as well.
On a TI 83/84 Calculator
Once again, we go to APPS and 1: Finance and 1: TVM Solver. The difference now is that our PMT is now positive because this is money coming from the account to our pockets. The FV will be 0 because our ending amount is nothing, we are emptying the account over the n periods. We put the cursor on which we want to solve and hit ALPHA-ENTER.
For the previous example:
| Enter the Information | After going to FV and ALPHA-ENTER |
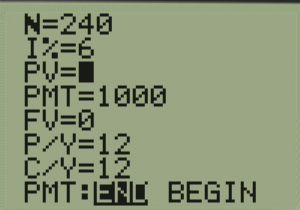 |
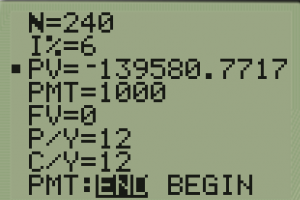 |
Notice that our starting amount we have in the account here is a little SMALLER than the answer we got by hand. Again, this is due to intermediate round-off error. Also note that it is negative because the money has to start in the account away from us.
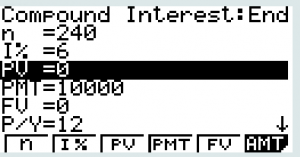
On CASIO
Again, this is similar to our procedure in the last section. Go to Menu-TVM and F2: for Compound Interest as it will allow us to put a PMT there. We fill in everything as before except we end with 0, so our FV or future value is 0 and PMT is positive. We use F3 to find PV or Present Value needed. (Note, if you do not see the bottom that shows what the function buttons indicate, try pressing EXE.)
For the previous example:
| Enter the Information: | After hitting F3 to find PV: |
 |
 |
In Excel or Google Sheets
In Excel or Google Sheets, there are several commands that figure compound interest for payout annuities:
- =PV(r/k, kt, w) will provide the P or present value needed, given the other variables
- =PMT(r/k, kt, -P) will provide the withdraw amount that can be taken with a start value of P for t years compounded k times a year.
- =NPER(r/k, w, -P) will give the number of periods the withdrawal can be taken, can be divided by k to get years.
- =RATE(kt, w, -P) will give the rate necessary to be able to take a withdraw k times a year out for t years out of $P.
For our previous example, we would type in =PV(0.06/12, 240, 1000)
Example Determining Amount of Possible Withdrawal
You know you will have $500,000 in your account when you retire. You want to be able to take monthly withdrawals from the account for a total of 30 years. Your retirement account earns 8% interest. How much will you be able to withdraw each month?
In this example,
We’re looking for ![]() .
.
![]() or 8% annual rate
or 8% annual rate
![]() since we’re withdrawing monthly
since we’re withdrawing monthly
![]() years
years
![]() we are beginning with $500,000
we are beginning with $500,000
In this case, we’re going to have to set up the equation, and solve for ![]() .
.
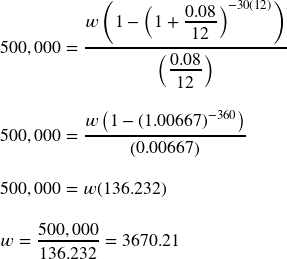
You would be able to withdraw $3,670.21 each month for 30 years.
Alternatively, you could use a calculator or Excel. On Calculator, the TVM solver would be filled in as:
N = 360
I% = 8
PV = -500000
PMT = 0 for now – or it doesn’t matter as this is what we are solving for)
FV = 0
P/Y = 12
C/Y = 12
END
On a TI: Cursor back to PMT and hit ALPHA-ENTER and PMT will change to 3668.822869… or in this case our deposit should really be $3,668.82.
On the CASIO: Hit F4 for PMT and it calculates the same as the TI.
On Excel: =PMT(.08/12, 360, -500000)
Note in Excel, we put .08/12 to keep the exact value instead of the round-off to avoid errors.
Try it Now
A donor gives $100,000 to a university, and specifies that it is to be used to give annual scholarships for the next 20 years. If the university can earn 4% interest, how much can they give in scholarships each year?
Try it Now Answers
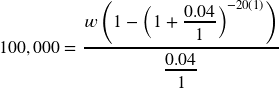 Solving for w gives $7,358.18 each year that they can give in scholarships.Using a calculator or Excel =PMT(0.04,20,-100000) yields the same results.It is worth noting that usually donors instead specify that only interest is to be used for scholarship, which makes the original donation last indefinitely. If this donor had specified that, $100,000(0.04) = $4,000 a year would have been available.
Solving for w gives $7,358.18 each year that they can give in scholarships.Using a calculator or Excel =PMT(0.04,20,-100000) yields the same results.It is worth noting that usually donors instead specify that only interest is to be used for scholarship, which makes the original donation last indefinitely. If this donor had specified that, $100,000(0.04) = $4,000 a year would have been available.Media Attributions
- 83example1
- 83example2
- 83example3
- 83example4

